So it was the Old Math Dog who pointed out that I never wrote a post explaining how I deal with the issue of kids not knowing basic algebra in calculus. I started this practice two years ago (when I also started standards based grading) and I have seen a remarkable difference in how my classes go from my life pre-bootcamps to my life post-bootcamps…
An issue in any calculus course — and I don’t care if you’re talking about non-AP Calculus or AP Calculus — is the student’s algebra skills. They might see and have no idea how to solve that. Or they might not know how to find
. Or they might cancel out the -1s in
to get
. It depends on where they are coming from, but I can pretty much guarantee you that every calculus teacher says the same thing to their classes on the first day:
Calculus is easy. Algebra is hard.
In my first three years of teaching calculus, I started with how all the books started, and all my calculus teacher friends started: a precalculus review. Then we went into limits.
The problem with that is that we might review some basic trigonometry, and then we wouldn’t see it again for months. And by then, they had forgotten it. And who could blame them. The precalculus review unit at the beginning of the course wasn’t working.
As I transitioned into Standards Based Grading, I looked at everything I taught really closely, and I honed in on the particular skills/concepts I was going to be testing. And since I’d taught calculus for a number of years prior, I knew exactly where the algebra sticking points were. Thus was born The Algebra Bootcamp.
Before our first unit on limits, I carefully analyzed what things I needed students to know to understand limits to the depth I required. I then looked at all the skills and thought of all the algebraic things, and all the old concepts, they would need in order to understand limits. And from that, I crafted an algebra bootcamp, and I made SBG skills out of just those limited skills.
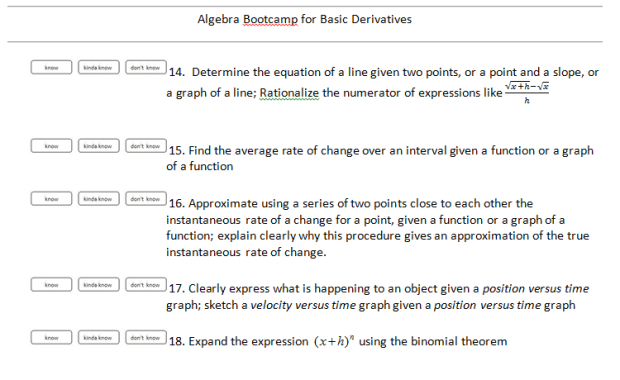
For example, here was our first bootcamp (which, admittedly, was longer than most of the others, because we were settling in and I was gauging where the kids were at):
and I did the same for other units… just the targeted prior knowledge that they tended to not know or struggle with…
Notice how they tend to be very concrete and specific? Like “rationalize the numerator” (because I knew we were going to be doing that when using the formal definition of the derivative) or “expand using the binomial theorem. Very specific things that they should know that they are going to be using in the following unit. It’s kind of funny because it is a hodgepodge of little (and often unconnected) things, and they have no idea why we’re doing a lot of what we’re doing (why are we rationalizing the numerator? why are we doing the binomial theorem?) and I don’t tell them. I say “it’s our bootcamp… once training is over you’ll see why these tools are useful.”
It is called “bootcamp” because I am not reteaching it from scratch. I’m reviewing it, and I go through things quickly. I only do a few of them in the first quarter and maybe the start of the second quarter. By that point, we’ve done what we needed to do, and they die off.
The reason that this has been so effective for me is because students aren’t having to relearn old topics/algebraic skills while concurrently learning the ideas of calculus. We review these very specific things beforehand so that when we approach the calculus topics, the focus is not on the algebraic manipulation or remembering how to find the trig values of special angles or what a piecewise function is… but on the larger picture…. the calculus.
Remember: calculus is easy, it’s the algebra which is hard.
So we took care of the algebra beforehand, so we can see how easy calculus is.
My kids in the past two years have made so many fewer mistakes, and we’ve been able to really delve into the concepts more, because I’m no longer fielding questions like “could you review how to do X?” Doing this has also forced me to think about what the purpose of calculus class is. The more I teach it, the more I take the algebraic stuff out and the more I put the conceptual stuff in. For example, I don’t use ,
, and
in my course anymore [1], because I wasn’t trying to test them on their knowledge of trigonometry. Doing these bootcamps coupled with standards based grading has forced me to keep my eye on what I really care about. Students deeply understanding the fundamental concepts of calculus. And I think you can do that without knowing how to integrate
just fine. [2]
[1] With the exception of for the derivative of
.
[2] I teach a non-AP calculus, so I have this luxury. But it’s nice. Each year I strip more and more stuff off the course and add in more and more depth. And I am glad that I understand depth to mean something other than “more complicated algebra in the same old calculus problems.”

Sam, This fall I’ll be teaching Calc I at the community college for the first time in about 5 years. I’m excited about your recent posts. You’re really helping me think about how I want to teach this course. Thank you.
Yaaay! In the next couple days I’ll be packaging up all my materials from this year and last year (I did some stuff exactly the same, some stuff totally different, and tweaked here and there, so both might be useful) to send to a twitter buddy who is going to be teaching calc for the first time… so if are interested, just shoot me an email to remind me, and I’ll copy you on that email too!
Hi, I am a first year Calculus teacher and have been looking at your blog all summer to prepare for ideas on how to teach my class! I LOVE what you have come up with. I just saw this comment that you sent files to a twitter buddy who was also teaching for the first time. Although this was last year, I’m hoping you still have some files you could forward to me as well? Let me know! I’d really appreciate it!! In the meantime, keep up the posts, I love to read them! :)
Sent you an email!
Hi Sam, I am in my 3rd year of teaching AP Calculus, and I am always looking for new ideas. If you are still willing to share, will you please send me your stuff?
Thank you!
Hey samjshah,
I am trying to prepare for calculus, and I was wondering if you can send me copy of of your materials? I will appreciate a lot.
Thanks
J
Hi! I am an AP calc teacher and have just run across some of your posts and love this. Do you share resources at all? I love the algebra bootcamp! If your materials are available, how can I find them? Thank you for sharing your great ideas and expertise!
Thank you.
Wow, what a great lesson for us all in the area of prerequisite knowledge. I really like how the bootcamps are purposeful. I can remember picking random Algebra review problems for students to do when I taught Geometry. Looking back, it was because I thought, “If only I could improve their Algebra skills…!” when I should have been targeting specific skills, a la Sam Shah.
Thanks for sharing. I enjoy reading your blog and realized that it’s been a while since I’ve taken the time to comment. Here ends the streak – blog on!
Love this. It is also a great roadmap for Algebra /PreCalc teachers who have not taught Calculus and maybe aren’t sure what the kids will struggle on (and need the most) going forward.
When you say, “I do a few of them”, do you mean you take a few days to do this? Do you do it all at once or do you break these days up (review a needed topic the day before you teach what the new topic)? Also, do you test them on Boot Camp concepts and give them boot camp homework? Sorry for all of the questions! I do this on a very small scale but would love to expand it.
Hihi… in calculus I take a few days to do the bootcamps. I do them all at once, before the unit. And I do give them light homework, and since I do SBG, I do test them on these topics.
But if it is thought out carefully, I think it could be done any number of ways. The first 10 minutes of each class, all at once before a unit, part of the nightly homework before a topic is used? To me it seems that you as a teacher just need to know precisely what skills/topics they should know but might have forgotten or what algebraic skills they are going to be using a lot but always mess up on year to year. If you have compiled that information, I suppose you can do bootcamps in any number of ways. I should have my kids say HOOAH! after we do each old topic, or something! Maybe an army chant? I don’t know, I’m not creative.
I should probably do this in physics…
Sam
I had the pleasure of teaching a non-AP Calculus class this year for the first time ever after 18 years of AB and 3 years of BC mixed in. At our school we made the decision to make this course strictly a differential Calculus course for the reasons you cite here. The kids in this course needed more time to renew their algebra/trig skills along the way and we did not feel the overbearing pressure of the calendar the way we do in AP. Also, I was able to maintain depth in a way that was similar to our AP class. These kids in my class did a little less in the way of ‘coverage’ of material but did as much serious thinking and problem solving. That being said, I know I’ll be tweaking this course next year to make it better and I would love to see some of what you have been doing if you don’t mind sharing.
Thanks!
This is a really great idea. I TA calculus at a big state university, and these bootcamps would help enormously. I just wish there were more time for things like this (that is, more discretionary time for us TAs to do something like this– everything is centrally coordinated, giving very little time for any sort of review)
Great idea indeed. Will definitely improvising my calculus course as well.
Thanks
I employed a similar idea for Pre-Calculus this year. Though I noticed a similar problem that everything was cool and useful when we did it but some of the boot camp lessons didn’t get applied until much later in the year. So next year I’m going to space it out more and probably make it a recurring topic on my tests.
I wrote about the subject and linked to all the worksheets I generated for it.
http://infinitesums.com/curriculum/?currentPage=2
I’d love love love to see that page you made (I’m teaching precalc next year), but the link doesn’t work. Le sigh.
I’m so jealous about teaching non-AP. I have to rush through so much material it’s crazy. Any chance you can post all of your algebra boot camps or just these two?
Turns out these are it (except for 2 trig skills – which I posted in a comment below)… You don’t need a lot of algebra/oldtopics to do calculus!
i would also love to peek at all your bootcamps (though something tells me they are on my comp since KSI). i’m going to go for this next year i think, and with my AP class too.
also, huge fan of eliminating the other trig functions and paring down the course. i have been battling with my dept head about this (though i really appreciate that she is keeping me up to a certain standard because otherwise my class would be a year of craziness). love teaching non-AP calc.
see you in a month!!!
They are def with the things I gave you at Kling…
I have to say, I have had ZERO issues with paring down the trig functions. I told kids they have to know how to calculate sec (because of the deriv of tan), but eliminating them did nothing to my ability to have students deal conceptually with what’s going on. If you ask “what’s the point?” a lot (like we did at Kling), you realize it just gets in the way.
Sam, I am going to be teaching the first Calculus class ever at my school this fall. (It is a very new school) I so want to get it started right, and I am very inspired by your posts. I would love to see the topics you chose for your algebra boot camps. I know you make them specific for the needs of your students, but a peek at your sequence would be a great framework to start from.
Actually, I thought I had one more, but the two images I have are pretty much it. I only do bootcamps in the first and part of the second semester. The one thing I didn’t put up there was trig basics — which I folded in a bit later. I asked for
1. Trig of special angles (only with sine/cosine/tangent), and graphs of sine/cosine
2. Sum of angles formula for sine/cosine (but I tied that bootcamp skill with the derivation of the derivative of sine/cosine)
Hope that helps!
Sam
Thanks!
Just seeing this now, Sam. I really like how you pare prerequisite skills down to the essentials necessary. I just finished my first year teaching, and of AP Calculus AB no less, with nearly 30 years between taking calculus and teaching it. Needless to say, last year was the most challenging experience in my life, and I’ve worked at some intense companies in complex fields such as wireless communications and GPS. Unlike your approach, I taught a week long, 2 hours per day, boot camp this summer to prepare students coming into my class this fall. While I narrowed it somewhat topically, I also touched on most, if not all, of the prerequisites they should be comfortable knowing prior to the first day of class. Even though I offered the bootcamp, I still plan to front load a mini-review of the necessary prerequisite skills just prior to introducing any new calculus topic this coming year. After one more year teaching the course, I hope to have a better view of the essential set of skills you’ve identified to make next summer’s bootcamp more effective, and increase the chance students retain that knowledge for the course.
Great job with your approach, and thanks for sharing!
Dave
PS If you are interested, here are two links to the boot camp and summer work for this coming year’s course. The first post includes results of a readiness test that I used to guide my emphasis in the boot camp.
http://mathequality.wordpress.com/2012/06/17/a-win-win-with-ap-calculus-boot-camp/
http://mathequality.wordpress.com/2012/06/24/atypical-ap-calculus-summer-work/
*forehead slap* This is brilliant! My school has a tradition of summer packets of work, which I mark in the first few days to assess which prerequisite skills each student struggles with. Then I review the most wide-spread problems, but all at once at the beginning. All while reading, I kept asking, “WHY didn’t I THINK of that?!”
Actually, that’s what I love about your blog (I’ve been lurking for about a year now, getting the courage to try SBG). So often you could be describing something that happens in my classes, and then offer some awesome piece of insight. Thank you so much!!
I’ve been a reader of your blog for quite some time but never commented. I teach at a 4-year college (Calculus I being one of the courses I’m teaching this coming semester). The “lack of algebra skills” is a real problem for most of my classes since my students can arrive in class via a variety of means (AP credits from high school, our pre-calc class, some other school’s pre-calc class, placement exam, etc.). One thing I’ve learned is that the universal algebra skills are quite poor – and your boot camp idea seems worth trying. I’m desperately trying to turn my Calculus I course into something other than “lecture, drill, repeat” like so many college courses… Of course, that requires both administrative blessings (of which I mostly have and am thankful for) and college students willing to “play along” which can be hit or miss depending on the semester/student.
Hello, I was wondering if you would be kind enough to send me the Calculus packet as well. I’ve been thinking of truly changing up how I teach Calc.
Hi Sam,
I taught non AP Calc two years ago, and will be teaching it again this year. I really like what you have with the algebra bootcamp. Would it be possible for you to post all of your algebra bootcamp standards? It’s something I want to adapt into my own class, and having an even clearer picture would be very helpful.
Sincerely,
Mark Perry
I am a new calculus teacher (non AP now) but will become AP next year. I have been following your blog and have found many useful things. I noticed you mentioned that you sent some calculus stuff to a few people and was wondering if I could be a part of the sharing?
Sincerely,
Diane Nurrenbern
Hi Diane,
You asked at just the wrong time. The service I use to share documents is going from free to paid, so I am shutting it down. Literally yesterday this happened! So everything I’m sharing will soon be not shared :(
Maybe I’ll have a new service set up soon where I will be able to share – I’m trying out a new one as we speak so hopefully that will work. For now, you can access all my posts re: calculus here: https://samjshah.com/tag/calculus/
This is going to sound weird, but if you reach out again in a month, I will probably have things more organized, and something new set up. I know I’ll forget so if you remember, please ask again!
Sam
Thank you! I will make a note and ask again next month! Any help is greatly appreciated as I muddle through teaching calculus for the first time since having it over 25 years ago!
Hi Sam, I’m reading through all the other posts and hoping that you have a new share method up now. I too will be teaching AP Calc for the first time next year (our school is adding Seniors next year) and was hoping you could send me your Algebra Bootcamps. I had the privilege of teaching these same kids in Alg 2, but they’ve spent a year in Trig/Pre-Calc & I know how much they will have forgotten. Your blog is very insightful! Thank you!
I know it has been more than a month but I am reaching out again wondering if you have a new “share” method! Any help is greatly appreciated!
Sorry it took forever for me to do this, but I FINALLY got my act together. If you happen to read this, let me know and I can see if my share method works for you?
Sam
I am reading it!!
Diane Nurrenbern Mathematics Teacher Gibson Southern HS On Jun 25, 2014 9:14 PM, “Continuous Everywhere but Differentiable Nowhere”
I’d be interested in your Boot camp file(s) as well!
I would be interested in your bootcamp material as well, if you are still offering it. I think your approach is inspired. Thank you.
I would love to get some material from you in preparation for my AP Calculus class next year. This year was my first year teaching AP Calculus AB and my students struggle with the foundational skills. Can you send me some material? I am already liking the idea of an AP Calculus Boot Camp which I would like to plan for next year. I look forward to receiving any information you have. Lamont Holifield
lholifield@urbanprep.org (Email Address)
I have enjoyed reading your SBG posts and your calculus posts. I was hoping you might share your bootcamp files.
Sam, I have just started teaching Calculus. It is a shame that I did not find your site sooner! Can I get a copy of the Algebra bootcamp? I also teach Analysis (for our school, it’s a feeder class for AP Calculus then next year), and I want to do a unit on Algebra-for-Calculus at the end of the year! Thanks!! =) bstalder@fgrhsaa.org
Hi Sam. I’m enjoying the material on your site. (It’s all very humbling.).
So, I am pursuing a math credential to teach all levels of high school math (and likely a masters in education as well), as a second career, after practicing law for 20 years. Ironically, physical disability (from a chronic heritable syndrome) created this opportunity for me to pursue my dream job. As I prepare for this test (mildly challenging for a non-math major, who never took courses beyond calculus), I am building a library of books and materials, to assist deeper understanding as well as for pedagogical purposes. To this end, I’d greatly appreciate a copy of your Algebra Boot Camp.
Additionally, I am wondering if you have any recommendations regarding materials to bone up on basic discrete math (primarily number theory) as well as modern abstract algebra (primarily fields and rings) — ideally something designed for someone who has not taken courses beyond a few semesters of calculus. For what it’s worth, I already acquired the U. of Chicago project’s precalc book (that includes an introduction to discrete math), as well as Dover on Number Theory, and Schaum’s Outline for Modern Algebra, the latter of which is a tad dense for me at this juncture.
Thanks much and keep doing what you’re doing!
I’m not Sam, but for the discrete math, I recommend the Art of Problem Solving books: Intro to Counting and Probability, Intro to Number Theory, Intermediate Counting and Probability:
https://www.artofproblemsolving.com/store/list/all-products
They are aimed at mathy high-school students and are more readable than most college texts, while being more rigorous than high-school texts. They also are good about providing thought-provoking, challenging problems rather than drill exercises. You can buy the solutions manual also, which gives detailed solutions to each problem—but don’t look at a solution until you’ve struggled with a problem for a few hours.
I agree with gswp above. I also recommend W.W. Sawyer for the Abstract Algebra. Here’s bookfinder.com. RIght now there’s a $7 copy: http://www.bookfinder.com/search/?ac=sl&st=sl&ref=bf_s2_a1_t1_1&qi=qZ413Xd9KVhsifWNzcKD9Msg3G4_1478547757_1:33:137&bq=author%3Dw%2E%2520w%2E%2520sawyer%26title%3Dconcrete%2520approach%2520to%2520abstract%2520algebra%2520dover%2520books%2520on%2520advanced%2520mathematics
Much appreciated, friend. Very generous of you to take them time.
I might have gotten some of that from “A Path to Modern Mathematics” (W.W.Sawyer) , though. (I’ll try to check at home later.)
Also, you might like The Art and Craft of Problem Solving, by Paul Zeitz, but it’s hard to find reasonably-priced copies of that.
Thank you for looking out, Sue.
Hi Sam,
I really appreciate your thought out algebra boot camp! Im teaching calculus for the first time this next semester and would really like to try doing things this way. Everything you mentioned are things I’ve been wondering about, and so I’m really grateful to find your page and hear your success story.
I would really appreciate if you could share any resources / the bookcase files with me if its still possible?
Thank you so much!
-Lucy
Lucillecourtney@gmail.com