General Ideas for the Classroom

A record of conference takeaways — TCM 2024

On Thursday, after school, I hopped in an Uber to the airport. I was flying to a conference, the “Teaching Contemporary Mathematics” conference (TCM) held at the North Carolina School of Science and Mathematics. I think I’ve been at least twice over my career, maybe three times, and always found it to be a really solid conference. The big sell for me is that it’s primarily high school math-focused, and most of the sessions are given by actual math teachers about their own teaching practice. And more importantly, it’s felt like forever since I just got to geek out with other math teachers. A fellow teacher from my school and I arrived pretty late and just passed out, with dreams of conference day on Friday.

Although I haven’t blogged in forever, I wanted to at least outline a few things tonight that I can return to. But it’s late and I’m tired, so I’m only going to do a few tidbits.

There were two sessions that got the mathematical side of my brain whirring.

Ryan P gave a talk on “A Rainbow of Random Digits,” where he went from 1D to 2D to 3D to think about a interesting problem. I thought what he showed was beautiful, especially for the 1D and 2D which I could see using with students in a sort of ongoing independent study/investigation. For the 1D question, he asked us if we had two dowels, of length 1 and length 2, and we split the larger dowel into two pieces, what is the probability that the (now three) dowels could form a triangle. A nice fun introductory question with various approaches to answering it. For the 2D question, he asked as a warm up: if you had two numbers chosen randomly between 0 and 1, (a) what is the probability that the sum is greater than the product, and (b) what is the probability that the sum of between 5 and 6? Lovely scaffolding, and the first question requires a little bit of calculus, which is fun. And then the full 2D question (which can be answered using some calculus, but I think it’s even more beautiful without it): if you have two random numbers between 0 and 1, what is the probability that the first non-zero digit of their ratio is a 1? a 2? 3?… 9? Totally fascinating 2D probability space, with lots of “triangle slivers.” To whet your appetite, here it is:

And totally not my intution, but the probability of getting a 1 (33.3%) is greater than the probability of getting a 2 (14.9%) which is greater than the probability of getting a 3 (10.2%), etc. The calculation involves some fun infinite geometric series. All of this was new to me. I also have no idea why getting a 1 digit is more likely than getting a 2 digit which is more likely than getting a 3 digit, etc. My intuition–which was way off–made me think all digits would be equally likely. Now honestly, I don’t know how teachers can actually build something like this problem into their normal classroom practice, but I do know that I’d love to work with a student to get them from the statement of the question to the answer in a lovely set of independent investigations, and some well-thought-out hints to guide. (And maybe chatgpt to write some simple code to do an initial simulation.) My friend and colleague suggested that maybe the distribution might be related to Benford’s law.

The second session that got the mathematical side of my brain whirring was by Bryan S. He had first learned about Conway’s “Rational Tangles” a few years ago, and wanted to present it to us. Wonderfully, I had first learned about these from Conway himself when I was in high school attending Mathcamp (and Conway was a guest lecturer). Conway was an electric speaker — and this one lecture of his imprinted itself on my mind. Now skip forward to this year. I had students work on “Explore Math!” projects and one worked on knot theory. I mentioned in my feedback to one student I had a really cool knot theory-adjacent thing I learned and I could show her. She responded saying “yes, please!” Of course it’s been years since I learned Rational Tangles. And it’s like the universe said “Oh, let me bring Bryan S. to you to remind you about all the nitty gritty of it.” And he was fabulous — a marvelous instructor who somehow managed to convey the excitement, weirdness, inquiry, all in a single short session. The crux of the setup is that you have two ropes held by four people:

There are two moves: T(wist) which has the front right person and back right person switch positions, where the front right person brings their rope under the back right person’s rope, and R(otate) where the four people just rotate clockwise. It turns out that by doing seqences of moves like this, such as TTRTRTTRT (etc.), you can get a pretty tangled tangle in the middle of the two ropes. One question — the main one we talked about — is if you can do a series of Ts and Rs to “undo” the tangle and get back into the original position of just two untangled ropes. Amazingly, a few Mathcampers created a digital version of this twisting and rotating and it took me about 20 minutes today to find it even though I knew it existed and I had played with it before. Here it is!

Another session I went to was Chris B’s Estimathon! I participated in my first estimathon at the Park City Math Institute many years ago (and again, a couple years ago). I hated it both times, for a few years. First, I hate estimating. I love thinking and calculating — but Fermi problems? I get really annoyed because I feel I rarely have the adjacent information that can unlock the problem. Second, the other people (both on my team and on all the other teams) were very, very competitive. I prefer a cooperative board game over a competitive board game, and in this, I think I feel similarly. That being said, I really enjoyed doing the estimathon with Chris and our other math conference participants. It was fun because the other people on my team were chill about it, and also let me sort of work alone at times when I got obsessed with a problem. Here are two example questions we were tackling:

Yes, I went to #5 because you can calculate that. Here’s what the scoring sheet looked like:

So you get to guess a minimum value and a maximum value for the range that the answer is in. And your score is ceiling(maximum/minimum). In other words, take the maximum value, divide it by the minimum value, and round up. So min: 2000, max: 3000 would yield a score of 2, and min: 2000 max: 4000 would also yield a score of 2…. but min: 2000, max: 4001 would yield a score of 3. Your goal is to get the lowest score. At the end, Chris gave us the absolute best idea (which I think he got from his colleague Emily). You have kids find out numbers for something that they are passionate about or would be an expert in. It could be “the number of pokemon” or “how many grandchildren does Sameer’s father have?” And then later in the year, you could create an estimathon out of these numbers — where kids have to see what they know about each other and their passions. I love this as a way for people to get to know each other.

There were two additional sessions that I attended, which were about students and math, and I loved both. First was by Jenny W, Lauren B, and Kevin J, and reminded me of the “5 practices” (https://www.nctm.org/Store/Products/5-Practices-for-Orchestrating-Productive-Mathematics-Discussions,-2nd-edition-(Download)/). It’s about using Desmos to highlight and discuss student thinking and to uplift student brilliance. Although I’ve seen many, many presentions on the 5 practices over the year (especially at PCMI), this was a great reminder of a lot of the things I don’t know, and teacher moves I’ve stopped flexing.

The second was by Lauren B called “I am, We are, You are.” It highlighted a few things to me. First, there’s a gap between the demographics of people who teach mathematics (and their identities) and the population of students who learn math (and their identities). She posed a question (of which she thinks the answer is yes): “Is there a way to expand identity in a math class?” I think this is a great question to chew on — and not easy. Especially if you take away classes like statistics or data science from the mix. We played with a super engaging Desmos activity which gave us choice on which data sets to plot against each other, and the fun part was guessing what the scatter plot would look like before we saw it. And she had a quote from Rochelle Gutierrez (who I’ve met before briefly!) which I couldn’t copy down quickly enough, but went something like “Do I have to be a better you in this classroom, or can I be a better me?” This is a student asking the question — essentially saying “Do I have to mold myself to be a miniature version of you, the teacher, to succeed in this room we’re in together?” I also thought that had a lot to chew on… in terms of what we expect from students and the culture that we build together.

Okay, it’s now 9pm and I’ve been at this for way longer than I intended, so to sleep I go.

***

It’s the next day and the conference has finished and I wanted to archive — briefly — the remaining sessions.

First, Hollylynne L. gave the keynote talk “Data Science is Everywhere and For Everyone.” Some takeaways for me are that there are a few large organizations (like NCTM, NCSM, ASA, etc.) that are collaborating to create a united data science position. It’s drafted, and about to be adopted, and has four guiding principles: (1) data science is contextual and multidisciplinary, (2) data science is an investigative process, (3) data science understanding and experiences are for everyone, and (4) data science educators must develop and practice ethical uses of data. The presenter shared her experience ethnographically observing data scientists for 9 months and what traits they exhibited. Then she shared with us that she feels like data science needs to be presented to kids with larger data sets — in terms both of cases but also in number of attributes shared (e.g. not just a survey with one or two questions, but a survey that has a ton of questions!). As an expert on CODAP, she shared how to fluently use it to show data, ask questions, and then interrogate the data. That part was inspiring, and in only a few minutes showcased the power of CODAP (codap.concord.org). Her talk also got me thinking about how our department has over the years shoved all of data science/statistics to our AT Statistics courses as we were making room for everything else we need to teach. Lastly, at the end, she shared a resource I want to follow up on called InSTEP. It is a free online site [https://instepwithdata.org/public/] that is designed to get teachers ready to teach data science and statistics, and it sounds like you learn lots of content, pedagogical moves, and you learn to use various tech tools but primarily CODAP (which is what I want to learn). So yay!

My next session was by Reed H and was an invigorating conversation on Standards Based Grading. He presented a “post mortem” of him implementing SBG in his precalculus classroom for the first time, sharing why he made the switch but also the tradeoffs that occurred. Although our school is moving in a different direction, I was still curious to see how the SBG conversations were going — and it reminded me how much I liked SBG even though it took me 4 years until I had refined it to the point where I could run it fluidly in my standard calculus classes. Reed’s own observations, and the conversations we had as small and large groups, also reminded me of my own path to SBG, and how I now know there is no single flavor of SBG that is going to work for all, because its success is dependent on so many cultural and institutional factors. And there is no magic bullet that is going to make it suddenly easy.

My last session was by Verónica Z and Doru H and was on Linear Programming and Other Means of Optimization. The presenters shared three ways to do optimization without calculus. First, the standard linear programming. The second was something called the “simplex algorithm.” Honestly, I got very confused at this point, because it isn’t in any way intuitive and I think that part of the presentation was meant for people who knew the method. So I ended up stopping my notes and writing “very confused” on my page. (I did find this example that went through the algorithm that I’m curious to read though, to get the crux of the algorithm, but don’t think it will explain “why” it works.) Finally, we learned the TOPSIS algorithm which was just invented in 1991 (“Technique for Order of Preference by Similarity to Ideal Solution”). New math! And the presenter, Verónica, did a really cool job of showing us how to make a decision of which new phone to buy (out of a choice of three) if we were looking at two variables: picture quality and battery life. It’s such a simple algorithm that — at the highest level — has you develop two new “fake” phones that exist — the best phone and the worst phone — one with the best of the traits of the three phones that exist and one with the worst of the traits of the three phones that exist. We plot all three phones and the best “fake” phone and worst “fake” phone. And then we find the “distance” from each of the three phones to the best and worst “fake” phones, and use those distances to rank the phones. Details are in my notes, but I loved learning new math in the universe, and new math to me!

Lastly, the conference was raffling off math art, and although I didn’t win, a new friend did, and she saw how much I coveted them and offered me hers. I demurred and then eventually accepted.

***

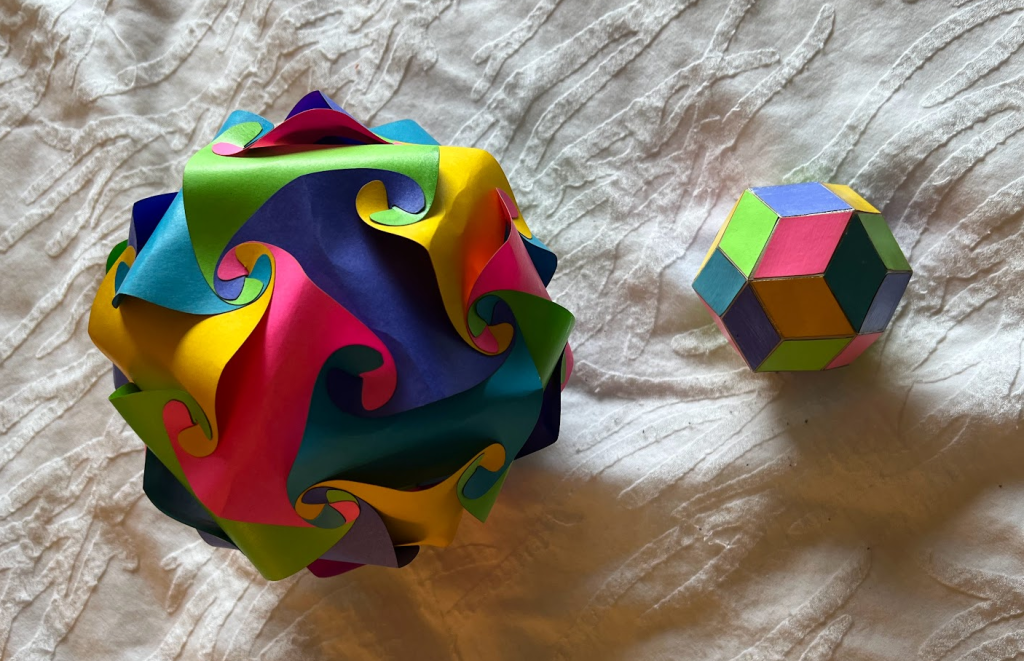
Personal Note: Speakers for the most part shared their slides with attendees. So I’ve downloaded the sessions I went to and saved them on my google drive. But I don’t know if they are officially public, so I’m just linking to them here for my own easy access.

Archiving an Idea for the Future: Reading and Writing About Mathematics

I had a thought that just occurred to me and I wanted to archive it before I forgot it. I’ll probably forget that it is even here at all, and nothing will come of it, but I had a thought about developing a new one-semester course for juniors and seniors at my school.

It would be called something like “Reading and Writing about Mathematics.”

I’ve always been obsessed with reading books that aren’t textbooks about mathematics. I have almost half a bookshelf filled with these books. I love (when I have time) reading articles about modern mathematics in Quanta magazine. I’ve sometimes formally incorporated reading books about math in my classes in the distant past (a variety of books and articles in multivariable calculus; a book called Weaponized Lies: How to Think Critically in the Post Truth Era in my Algebra 2 class). And for many years, I’ve reached out to kids and set up many math book clubs, where we meet over lunch for a few different times to read books about math. I even was once interviewed about this, and wrote an article about this.

So why not formalize this into a class?

The biggest benefit I can see for the class would be introducing students to what modern mathematics is, so they don’t leave thinking mathematicians are just doing precalculus or calculating integrals in their ivory towers. Just like kids in physics will learn that the physical world is weird and wild when they are introduced to the ideas of quantum mechanics and relativity (even though they aren’t delving into the nitty gritty) in high school, I’d like a way for kids taking this class to learn that the mathematical world is weird and wild… and most importantly: human. A course like this will humanize math for students.

Right now I’m reading Jordan Ellenberg’s book Shape: The Hidden Geometry of Information, Biology, Strategy, Democracy, and Everything Else [here], with 5 students and 2 other teachers. As we’re reading it, there are parts that intrigue students but we need to parse it out together for it to fully make sense. One example was in this book, Ellenberg talked about the question “How many holes does a straw have?” and he brings us into topology and understanding how holes can have various dimensions. As we parsed this, I thought: oh, reading this and then also doing some mini-problem sets on the math could help kids understand things at a deeper level, or confirm their understanding of what they read. We could do our own simulations of random walks, in the section involving that idea. We could parse out the idea of the “necklace problem” which we’re going to talk about at our next meeting, which I’m sure the kids won’t understand because they aren’t drawing things out but more passively reading. In other words, we’d be able upgrade a passive reading of the book to an active reading of the book with a mini-problem set that brings ideas that might not fully make sense to life.

Additionally, there are books or articles that we could read that would help students understand the social construction of mathematics (hello anything on the controversy of the ABC conjecture and Mochizuki’s “proof”).

We could learn about the origin of ideas that seem to have existed forever or that we take for granted, but actually had to be developed (e.g. the idea of “0” or the controversy over calculus being on firm footing). And mind-blowing ideas like how all of mathematics almost fell down with the work of one mathematician, Godel. And we can learn about marginalized and overlooked people. There are some really great children’s books on mathematics, so we could read some of those as we begin the class! Maybe complement that with some excerpts from Douglas Hofstader’s Godel, Escher, Bach, a newspaper article about a modern mathematical breakthrough, some math poetry, a formal mathematical paper that has come out in the past 10 years and is hailed as one of the most important discoveries, and one more piece that’s maybe more traditional, so kids can see the wide variety of ways people write about mathematics.

And kids would think about how the writing that they’re reading is effective to reaching their intended audiences, or how it isn’t effective and what would make it better.

And kids could pick ideas or people to learn about, do their own research, and write their own popular mathematics writing. We can workshop it and publish a little journal at the end of class with our pieces.

I could reach out to English teachers to learn how they facilitate conversations about books so that it doesn’t go stale (we aren’t doing the same thing every time we discuss some of the reading). I’m sure there are universities that offer similar classes, so I could see what else is out there. And I know MIT has a graduate program in science writing that I could draw ideas from.

Okay, I’m done brainstorming for now. I just wanted to get all these ideas out before I forget them. I might update this post with additional ideas as my mind percolates. As I said… most likely nothing will come of this. But I could see having a really fun summer trying to put this course together.

NOTE: The deadline has passed to propose this course for next year. But it would take me a long time to develop the course outline anyway, so I could try to design it this upcoming summer and submit it for the following year.

I’m not sure this is comprehensive, but this is a list of books I’ve worked with kids/faculty in various capacities on [but it is not all the popular math books I’ve read]

*=I’ve done this for a student (or student and faculty) book club
**=I’ve led/organized a book group with just teachers on this
***=I’ve had students individually read this (to discuss with me in an independent study or for a math project)

*Anna Weltman, Supermath [here]
*Edwin Abbott, Flatland [here]
**G. Polya, How to Solve It [here] 
*Amir Alexander, Infinitesimal [here]
***Charles Seife, Zero: The Biography of a Dangerous Idea [here]
*G.H. Hardy, A Mathematician’s Apology [here]
***David Leavitt, The Indian Clerk [here]
*Steven Strogatz, The Calculus of Friendship [here]
**Steven Strogatz, Infinite Powers [here]
*Edward Frenkel, Love and Math [here]
*Robert Kanigel, The Man Who Knew Infinity [here]
***James Gleick, Chaos [here]
***Yoko Ogawa, The Housekeeper and the Professor [here]
*Margot Lee Shetterly, Hidden Figures: The American Dream and the Untold Story of the Black Women Mathematicians Who Helped Win the Space Race [here]
***Hiroshi Yuki, Math Girls talk about Integers [here]
***Hiroshi Yuki, Math Girls [here]
*Jordan Ellenberg, How Not To Be Wrong: The Power of Mathematical Thinking [here]
*Jordan Ellenberg, Shape: The Hidden Geometry of Information, Biology, Strategy, Democracy, and Everything Else [here]
*Daniel Levitin,Weaponized Lies: How to Think Critically in the Post-Truth Era [here] — the first third
***Ben Orlin, Math With Bad Drawings [here]
*Ben Orlin, Math Games with Bad Drawings [here]
***Paul Lockhart, A Mathematician’s Lament [here]
***Cathy O’Neil, Weapons of Math Destruction: How Big Data Increases Inequality and Threatens Democracy [here]
*Hannah Fry, Hello World: Being Human in the Age of Algorithms [here]
***Allison K. Henrich, Emille D. Lawrence, Matthew A. Pons, and David G. Taylor, eds, Living Proof: Stories of Resilience Along the Mathematical Journey [here]

PCMI 2022 Post 4: Assessments

At PCMI we’ve been talking a lot in our “reflection on practice” sessions about assessments. Starting broadly, we wandered in the realm of epistemology and started talking about what we mean by knowledge, and how we know if we know something. Which then led to conversations about how we have evidence of knowing (and why it might matter that we teachers think about this and reach a consensus on this).

From this more abstract beginning (where we did try to bring it down to the classroom level), we then started to get more concrete. A very generative question was “what does it mean to be mathematically competent/proficient?” Everyone interpreted the question in a different way, and initially I was stuck – do we mean in a particular skill? or overall, when can we call a person’s body of work “mathematically competent/proficient”? procedurally or conceptually? isn’t competent < proficient? So many layers to unpack in the question itself. However I still started brainstorming and the very first thing I came up with was “can see the idea in a larger web of ideas/connections.” I often think that’s a hallmark of a strong mathematical thinker – where things aren’t this hodgepodge of ideas and procedures but they are tied together in a larger web. And what’s lovely is that one of the most powerful parts for me of Francis Su’s Mathematics for Human Flourishing book so far was in the Meaning chapter:

mathematical ideas, too, are metaphors. Think about the number 7. To say anything interesting about 7, you have to place it in conversation with other things. To say that 7 is a prime is to talk about its relationship with its factors: those numbers that divide evenly into 7. To say that 7 is 111 in binary notation is to have it dialogue with the number 2. To say that 7 is the number of days in a week is to make it converse with the calendar. Thus, the number 7 is both an abstract idea and several concrete metaphors: a prime, a binary number, and days in a week. Similarly the Pythagorean theorem is a statement relating the three sides of a right triangle but it is also, metaphorically, every proof you learn that illuminates why it is true and every application you see that shows you why it is useful. So the theorem grows in meaning for you each time you see a new proof or see it used in a new way. Every mathematical idea carries with it metaphors that shape its meaning. No idea can survive in isolation — it will die. (37) [italics mine]

In addition to this being part of being mathematically competent (which, overall, I think I ascribed to a body of work that a student did instead of with a single skill), I also immediately thought of various mathematical habits of mind.

Something that struck me, after my brainstorming, was that much of what I wrote was about a world of ideas, but it was disembodied from the physical world… and so I ended my brainstorming: “One thing I’m thinking about when I think about “mathematical competency/proficiency” is that those terms seem to be pretty clinical… I’m wondering if joy and appreciation and an emotional connection to math would exist in this or not.” Looking back at this reflection of mine, I think of Rochelle Gutierrez’s eight dimensions of rehumanizing math, and my wondering seems to fit squarely in the “Body/Emotions” dimension.

As I’m writing this blog post, I’m enjoying seeing how lots of different ideas in this conference seem to all be strung together loosely and I’m only now seeing them braid together.

One great exercise we had was just writing down all the different ways we collect evidence of student understanding. All the ideas we came up as a group are here, and a word cloud of what we came up with is:

We talked about bias in various ways we collect and interpret evidence, the ease/difficulty of various ways we collect evidence, and how confident we were in our interpretation. What I liked about this is that we went beyond “paper tests” and “exit slips” and allowed the true range of things that we as real teachers do to get a sense of what a student or class knows… I mean, I do actually use facial expressions to help me get a sense of student understanding, but I also recognize it’s not always the best indicator. So this broad list we generated didn’t seem like a trite exercise because it valued all the ways we as teachers do truly get a sense of things on a day-to-day basis. In other words, it broadened our sense of “assessment” to go beyond “the things that we grade, that often are done on paper.” Assessing is just getting a sense of what kids know – whether formative, summative, or something else (e.g. self-assessing their own confidence on something).

We read a couple articles on assessments as well as having kids self-assess or peer-assess. I liked NCTM’s section on assessments from their Principles to Action — reframing assessments to be something that provides feedback to students but also informs instruction moving forward.

Shifting the primary focus and function of assessment from accountability to effective instructional practice is an essential component of ensuring mathematical success for all students (p. 98)

For me, the big reminder was that we teachers traditionally think about assessment as a noun. That paper thing we give. A static snapshot. But we should think about it as a verb, assessing, and that is part of a learning cycle, a journey — both for student and teacher. However, of course, there’s the ideal and there’s the reality.

There are ways I’ve thought more as a verb, like when I’m did standard-based grading when I taught calculus. But usually, when I give summative tests (which is what I normally do, and I don’t really do projects), I don’t think of them as a part of a cycle or journey. They are the static snapshot.

But I also know in reality, I do lots of assessing in small ungraded ways (self-assessing, start of class problems and walk around, listen intently to students talking, looking at nightly work, etc.), and use that to inform my next teaching move or plan my next class. For one example, I have a general idea of what the nightly work will be each day, but based on what I see in class, I often will alter it based on what makes sense… or generate some new problems to address a misconception or gap I’m seeing… or to have students think about a particular insight that came up in class.

I’m now getting tired, so I want to end with three things before I lose steam.

First, we watched a video of Max Ray-Riek on why 2 > 4. It is an ignite talk, and I’d seen it before, and I realized after watching it that during then pandemic, I started listening for instead of listening to because I felt so stressed for time. I think this is a video every math teacher should watch!

Second, we brainstormed ways that we get real-time evidence of student understanding in our normal everyday classes. And then we each chose a few to share out. I like this document of collective knowledge that we generated, and I want to come back and read through it again to be inspired.

Third, we talked about ways we had students self-assess themselves and their own understanding as a way to become more independent learners, and think metacognatively. I really enjoyed brainstorming this individually and listening to everyone else’s ideas. I wish we had more time for this activity — because I love talking about concrete things we do in the classroom so I can get more ideas and rethink things I already do!

And with that, I’m done! Tomorrow begins our third and final week at PCMI!

PCMI 2022 Post 2: 3D printing

I was so grateful to the neat ideas that I got on twitter about 3d printing, which I included in my last post. (If you want to check the tweet and the replies, they are here.) Some of the responses that really stood out to me are here:

(1) Have students design (using their knowledge of some core functions and transformations) bubble wands using Desmos. Ashley Tewes wrote a moving blogpost about it here (and how she tied it in with empathy and a larger audience than just the students). And just look at how fun and beautiful these are!

And in a similar vein, Martin Joyce has kids use Desmos functions to create objects involving their own names! And @dandersod showed how to 3D print a polar graph from Desmos to be an ornament, which I then did on our school’s 3D printers:

… and I was going to have my kids do our polar graph contest and have the winner’s graph get 3D printed (but the designs were too intricate for that, sadly).

(2) Kids can design their own tesselations (learning the ideas behind how various constructions can build tesselations) and then create 3D printed cookie cutters for them — so they can create “cookie tiles” that tesselate! Or penrose tiles! I initially found a neat blogpost which I’ve lost, but here’s a tweet that showcases it!

(2) Mike Lawler has almost a hundred posts where he and his two sons (who do math together for fun) have used 3D printing. And they are all pretty dang fabulous — an amazing resource. He even chose his favorite ten 3D printed projects here if you don’t want to scroll through all of his posts. The last one he listed in his ten was a model that illustrates Archimedes’ method for deriving the volume of a sphere (without calculus)! I remember learning this in high school and was blown away (so unexpected! so beautiful!), but in all my years teaching, I had never seen this particular manipulative. You can see and download the manipulative here, but I’ll throw down a screenshot of it:

(3) When I taught Multivariable Calculus, we had talked about mappings and coordinate systems, and so one year a student 3D printed this stereographic projection (among other things) and then wrote a paper which analyzed how this all worked:

And I remember showing my multivariable calculus students, in another year, a bunch of optical illusions made by Kokichi Sugihara. They blew my mind, and the kids were smitten. One read some papers on the math behind how you can design these and wrote up a cogent explanation of how this worked using a neat analysis of vector-valued functions.

And goodness knows 3D printing is so cool for surfaces in multivariable calculus, and so much in regular calculus.

But I have to say: after doing a lot of sleuthing, getting things sent to me by others, and just trying to wrack my brain, I’m honestly pretty disappointed with what I think I can do with it in the classroom. It might just be me, but all these schools a decade or so ago were like “WE NEED THESE 3D PRINTERS BECAUSE THEY ARE GOING TO REVOLUTIONIZE STEM EDUCATION.” Maybe so. But after doing an initial foray into them, my current thoughts are: pfft. Maybe I’ll change my mind, but right now: pfft.

Right now, for me, I see the value in 3D printing in two main domains:

MANIPULATIVES: So as I noted, in my last post, there are tons of cool manipulatives a teacher can find and 3D print to illustrate an idea. Like the Archimedes’ proof for the volume of a sphere, or the optical illusion, or creating penrose tiles or printing many of the 15 pentagons that tile (so kids can fit them together and play!), kids will learn. They may be captivated. But kids are learning just from the manipulative, not from the process of 3D printing. That’s just the point of the manipulative — and the 3D printing is one way of getting the manipulative. So great. It isn’t the process of 3D printing that drives student understanding, it is just the manipulative that the teacher finds to illustrate the idea, that happens to be a 3D manipulative. And that’s cool. There’s some value. But in the same value that you can open any math teacher catalog and find lots of hands-on things for kids to play with. This is just a 3D printer printing them, instead of ordering them.

OBJECTS TO SPARK JOY, BUT DON’T HEIGHTEN MATHEMATICAL UNDERSTANDING: Then there are things that I think kids would love doing with the 3D printer in a math class… building bubble wands by using Desmos and function transforemations… developing cookie cutters by learning about transformations… creating polar ornaments by designing creative and beautiful polar graphs. Kids will be able to hold their creations, feel an ownership of mathematics, be proud! So I think there’s a lot to be said for these types of activities. I want to do them! But at the same point, I also truly feel like all the conceptual mathematical learning is happening before the 3D print. The 3D print doesn’t do anything to build on that understanding. What does printing the polar graph ornament from the 2D Desmos polar graph actually teach kids in terms of math? Nothing. I’d argue a kid who printed their bubble wand and a kid who didn’t probably learned the same things. Yes, these things are dang cool, so there’s something to be said for that, but I would argue they don’t build student understanding.

I posited in my last post that there might be a third domain where 3D printing is powerful: where the act of kids actually doing the building in tinkercad or whatever software builds conceptual mathematical understanding. This has been my unicorn, the thing I’ve been really trying to think about or find in the past few days. Because if I’m going to have kids spend time learning new software and troubleshooting finicky 3D printers, there better be a big learning payoff. But at least for Geometry, Algebra 2, and Precalculus, I have yet to anything that really fits the bill.

So for now, as a teacher, I say “hey, 3D printing is cool, but overall, pfft.”

(You might feel differently about this and that’s cool. And I might change my mind. But since I’ve been sending a lot of time trying to think about this and look stuff up, I have just felt a lot of disappointment when I was hoping there was a lot of untapped promise.)

Mathematical Habits of Mind

I haven’t been blogging for a long time. As you can imagine, the pandemic took a toll on teachers, and at least for me and my teacher friends, we were working insane amounts of time, and it was so hard. Emotionally, physically, intellectually. At the time, I just didn’t have it in me to blog about the experience.

But now we’re about to start a new school year. And I’m vaccinated. And my students are vaccinated. And we’re wearing masks. And my classes are going to be with all my kids together in a single room [1], which is such an awesome thing compared to last year.

One of the classes I’m teaching this year is Advanced Precalculus. Another teacher, my friend James, is also teaching the same course. And he’s new to my school this year, and so when talking about the course, he shared with me how he formally incorporated Mathematical Habits of Mind in his teaching in previous years. And interestingly, last year, I toyed with the idea of formally getting kids to be metacognitive about problem solving strategies — but decided to focus on something else instead. So when James shared this idea with me, I got excited.

Right now I have an inchoate idea of how this is going to unfold. Hopefully I’ll blog about it! But for now, I wanted to share with you posters I made using James’ Mathematical Habits of Mind. Most importantly, here is a link to James’ original blogpost with his habits of mind and rubric.

Photo of the posters hung up in one of my rooms:

I know, I know, the lighting is terrible. The key words are:

Experimenter, Guesser, Conjecturer, Visualizer, Describer, Pattern Hunter, Tinkerer, Inventor

If you want these posters, the PDF file is here.

mathematical-habits-of-mind-postersDownload

And here are all of them shared as a single sheet, and not as a poster.

one-sheet-mathematical-habits-of-mindDownload

Of course, if you’re a math teacher, you know there are a lot of lists of mathematical habits of mind. We agreed to use the ones James had already been using. But there are many alternative or additional things we could have included.

At the very least, I know that as we get kids to think about what strategies they’re using to solve problems, we’ll also see where there are lacuna in our curricula in terms of using those strategies. Or maybe we’ll discover it doesn’t have as much problem solving as I imagined in it. All entirely possible, since we — the kids and James and I — will all be looking through what we’re doing through our metacognitive Mathematical Habits of Mind lens.

[1] The reason I note this is because at the end of last year, I was teaching students live simultaneously in three places: they were in two different classrooms and there were a few at home on zoom. Yes, seriously. When I mention that to teachers and non-teachers alike, they asked how that was even possible. It was… a lot.

How I Schooled During the Spring of 2020

I kept on wanting to write up a short post outlining how I dealt with online teaching in the Spring and reflect. But the year ended with a bang, and I wasn’t in a headspace to do this. I’m going to do that now, but without too much reflection, since now there’s too much distance — the details are lost.

What I can say is that I did similar things in both Algebra II and in Advanced Precalculus. I would say based on the regular feedback I was collecting that all students really appreciated my organization, consistency, and clarity. That being said, even though I had pretty much the same structure in both classes, things seemed to go better in Advanced Precalculus. I have some conjectures as to why, but I don’t really know the cause.

 

Update: A Disclaimer and Caveat

I wanted to write this up for me, to archive my process/thinking. It helps me. But I also want to make clear that this isn’t a how-to guide for anyone else. This is just how I figured out things to work in the situation I was in. In teaching, context is key. There is never a one-size-fits-all approach. I have so many friends who had to teach “but not any new content” or weren’t allowed to expect that kids would be able to join class “live,” or had to do everything asynchronous. Of course much of this wouldn’t work in many situations. And more importantly, I have so many friends who have kids or other obligations that took up much of their time. If I had, for example, a kid, I would need to come up with a totally different plan. To be clear, this was not sustainable for much longer. It worked for me for three months, hopefully for my kids, and I say overall it went “fine.” But I couldn’t do this over the course of a year. I didn’t talk about my mental state in the original post because I did a lot of that processing with friends in the moment. But let’s just say this whole sudden online teaching took its toll. There were so many evenings I wanted to break down and cry. I was frustrated, mad, angry, overwhelmed, drowning, and felt like no one could really understand. Like this was a Sisyphean task. If I shared with you some of the texts I wrote to friends, I’m guessing even though we were in different situations, you would totally point and say “yeah… me… that was me.”

 

The Planning

I spent a good part of my Spring Break trying to envision what class online could be. I used my friend Alice as a sounding board and I realized I had to figure out what my core values are that I wasn’t willing to compromise on — and build from there in the space we had available. I hit on these three things:

5Initially, we were given very short classes (30 minutes) and then later they were extended to 35 minutes.

 

The Setup

I opened a document to write a revised set of course expectations. And as I thought about each section, I started to be forced to imagine what our class was going to look like, how students were going to be assessed, how I was going to make things manageable for me, how I was going to provide support for my students. I didn’t quite know what to expect before we entered this phase. It could have been only for a few weeks, or (as it turned out) it could last to the end of the year.

Here’s what I came up with:

Online Learning! – Adv. Precalculus – Google Docs

The main highlight of this is that I switched our courses to Standards Based Grading. Our school went to Pass/Fail for the second semester and I wanted a way to assess that would support my kiddos. This also gave me a way to determine Pass vs. Fail. I’m really familiar with making SBG work because for years I taught standard Calculus and I learned how to change the flavor of SBG so it worked for me in my particular school.

Most importantly, although we switched to virtual school, my goal was to keep our classes as consistent as possible in terms of how students would learn. I didn’t want to immediately make students work individually since they were used to collaborating in teams. I didn’t want to give them videos showing them how to solve some sort of mathematical problem since they were used to figuring that out themselves.

Everything wasn’t perfect, but I can say that overall the feedback was pretty positive. Here are a few comments from a reflection/feedback form I gave to kids a few weeks into our online learning:

Honestly, it’s working so well for me. This class feels the most structured and like I’m engaged and getting something out of each class. Thank you for all the effort you put into making the Demos activities!!! I also really like the structure of watching videos outside of class, and then coming back to any questions and building off of what we watched.

I personally find that math class is working really well for me. We’ll see how the upcoming assessment goes, but I feel like I’m understanding the material we are being taught almost as well as I did in live school.

I think the structure of our virtual math classes is pretty successful. I really enjoy working in breakout rooms together with my classmates. It allows me to “spend time” with people and work on math together, which is awesome. I also really like when you spend a few minutes explaining concepts by sharing your screen and using a virtual whiteboard. It feels pretty close to the normal organization of our math classes.

 

The Constraints

Our schedule allowed us 30 minute classes for the first few weeks we did online learning. Then, when we refined the schedule, we were given 35 minute classes. They were short.

Almost all of my kids had working internet and a school-issued laptop. Access wasn’t a huge problem.

I decided I had an obligation to hit all the major ideas I would have covered, but I felt comfortable paring things down to smaller and more essential bits, and eliminating the things that felt more minor.

We have a weird 7 day rotating schedule where we had 5 times we could meet. We had a choice for how many of those classes we wanted to be “live.” (Some teachers, like history or English teachers, would have kids read or work on papers for some of the days and then meet live only a couple times each cycle. Or they would set longer one-on-one meetings up with their kids to talk through ideas.) For the structure I set up, I usually had my kids meet “live” 4 out of every 5 classes — and I had something for them to do that didn’t require collaboration for the fifth day. It’s important to note that these classes were a mere 30 minutes (later extended to 35 minutes), so every minute was precious.

We were using zoom as our communication/video platform.

I didn’t want to give a lot of nightly work, since kids were going to be on their computers a lot. Since we were meeting live a lot, my goal was in the range of 5-30 minutes, depending on the day and their level of understanding.

 

Organization

I used the “Classwork” tab on Google Classroom to be our central hub. At the top of the page was:

10

I had a revised version of our course expectations, an ongoing skill list for what we were learning, and a link to my google calendar where kids could reserve a time to meet with me individually. (The other links aren’t as important.)

Then below that I created a different “topic” for each week of learning:

9

We were asked to create an assignment for each live class meeting we had — so that it would appear on student’s google calendars (since we had the option of holding a live online class or not). Then each day I would add the nightly work. Notice I would have the nightly work due by 7am the next day we had a live class. I’ll explain why that was so below.

 

The Planning

Here’s how it worked. I centered the learning using Desmos Activities. I didn’t want kids to have to learn a new platform (they had used Desmos Activities a number of times before). And Desmos had instituted a way to give students feedback.

So the crux of every live class was students working on Desmos Activities that I had adapted or created from scratch. They worked together in breakout rooms, where one kid would share their screen and they would work through the activity together. Some of the slides were “practice” — so not much talk would happen — but some of the slides included exploration and investigation and conjecturing and explaining conceptually what’s happening.

Here are all my Desmos Activities for Advanced Precalculus used during remote learning: https://teacher.desmos.com/collection/5e80e25ec9089c33af3d954f

Here are all my Desmos Activities for Algebra II: https://teacher.desmos.com/collection/5e80e247431047086cf42c54

I kept two evolving separate google documents with my lesson plans for each day. They looked something like this — with easy access to links that I could copy and paste quickly into the zoom chat box when I needed them to go to an activity.

6

7

I’m a teacher that likes to go at the pace of my students — so my different sections weren’t always perfectly aligned. I would design the next class based on where kids got.

Here’s what a “normal” class might look like from a student perspective (remembering we only had 30 or 35 minutes):

  1. Kids join the zoom. Near the end of the year, they started hearing me playing music as they were admitted into the class. It gave me something to bop along to and put me in a good mood! :)
  2. Kids hear me say “hi!” I send kids (in the chat box) the link to the Desmos Activity they had been working on and ask them to go there and spend a couple minutes silently looking at the feedback I left them.  I do this for just a couple of minutes — most of the feedback is short, and I tell them to look more seriously at it after class. We don’t have much time together.
  3. Kids hear me outline what I took away from the work they did during the previous class and what they for nightly work after the class. If there were issues that more than a couple kids in the class had, I made sure to address it in the whole class. I would do this by screensharing a particular slide of a desmos activity and talk through it, or sharing my iPad and talking through an idea. During this time, I might occasionally preview an idea or remind students of something they had seen previously that might come in handy. This would take 2-7 minutes. (But with 30 minute classes, I wanted to have kids work together during the majority of the time.)
  4. Before kids go to the breakout room, they hear me say: “Okay, you’re going to log into this Desmos activity. Write this down in your notebooks — today you’re going to call me over so I can talk with your group at Slides X and Slide Y. Remember if I’m busy to keep working and I’ll come by when I’m done with the group I’m with.”
  5. Kids work together in their breakout rooms. Sometimes they’ll see me pop in when I’m following along on their work on Desmos and see something I want to point out, correct, or compliment. (I didn’t have much time to compliment, honestly, though I tried to do that so me popping in always didn’t seem like it would be a critique.) When they get to particular screens where they were asked to call me over, I’ll join and give them feedback, ask a few questions I’ve prepared to assess they know what they’re talking about, and then have them contiinue on (or ask them to discuss more after I nudge them forward, and then call me over if they didn’t seem they got an idea).
  6. Three minutes before the end of our time together, I’ll either send kids in breakout rooms a message saying they can leave at the end of the class straight from the breakout room, or I’ll call them back to the main room to say something and then dismiss them.
  7. The nightly work will be posted on google classroom pretty soon after class. The assignment will look like this:8I’ll ask them to review my feedback from the previous night’s work some more. Sometimes I share with them a resource if they struggled with that work (usually a video I created going over some of the problems.) I post what they’re supposed to do. Sometimes I’d include DeltaMath practice for more routine problems, which I love because it gives students feedback on how they’re doing.

On my end, this is what my side of things look like to make this all happen

  1. School starts at 9am, so I wake up at 7am and shower and get ready by 7:30am. I sit down at my table and look at my calendar. I tell me Amazon Echo to send me reminders 5 minutes before every class/meeting I have.
  2. Then I look through the Desmos activities for the classes I was seeing that day. I always made the nightly work due by 7am of the day we’re having class, so I could look it over and understand where kids were at, and give them immediate feedback on their work/thinking. I go over every student’s slides (choosing key slides to give feedback on). As I do this, I make a note of which topics are worth bringing up in class — if anything. There were a good number of days when kids seemed to get most of the material!
  3. As I do this work, I also fill in a nightly work spreadsheet to keep track of whose doing the work. I also had a column where I started keeping information that might be useful about things I noticed in their work, but truth be told, keeping that additional column wasn’t sustainable so I ended up using it for notes about when kids didn’t do their work — if I emailed them, what they said, etc. (As a side note, if a kid didn’t do their work, I let them complete it later.) Here’s a sample of what my spreadsheet looked like.
    12
  4. If I see a bunch of students are struggling with an idea, I quickly prep a short iPad presentation to talk about a concept or work a problem — a mini-lecture I’m going to deliver. I add that into my lesson plan for the day. From start to finish, looking through the nightly work for the kids and doing any last minute mini-lecture prepping usually takes me a little over an hour.
  5. I open the classroom 5 minutes before class starts. As I admit kids into the zoom room (two or three at a time), I mark them present on my attendance spreadsheet. Sometimes when kids come too quickly right at the start time of class, I’ll just admit everyone and fill this in when kids are in their breakout rooms. (This is a fake spreadsheet to illustrate.)11
    When everyone has arrived, I say hi and then tell kids to check the feedback I left for them on their nightly work (on a Desmos activity). I put the link in the chat box.
  6. After they look at the feedback, I gather us together. I go over the things I noticed from their work in the morning, and give any mini-lectures I feel is necessary for that section. I send them off to work on the Desmos activities — telling them to call me over at one or two pre-chosen slides. Usually, I set them up in random groups of 3-4 students, though occasionally I’d do pairs for certain activities.
  7. At the very start when they’re working in breakout rooms, I’ll take a piece of paper and write down the answers for each slide I think they might get to. That way when they enter their work into desmos, I can quickly check it. This usually only takes me a few minutes and kids are still settling into working together.
  8. Then I start keeping tabs of what’s going on by using the teacher dashboard. I can see which group is on which slides. I write down on a piece of paper the name of one student per group, and I tend to follow along using that student’s work as a representative for the group. As they’re working, I’m noting down which slides they’ve completed correctly and if there is anything I need to talk with them about when their group calls me over. I’ll occasionally pop into breakout rooms when I see a group is stuck and needs some help.
  9. After groups get to a particular slide and call me over, I’ll look over their work (if I wasn’t able to keep up as they were working) and ask them questions I had pre-scripted to check their understanding. I ask if they have any questions for me, and then they go on. These pop-ins are short — as short as a minute, but if we start discussing, we can get to three or four minutes.
  10. I’m following along and checking in for pretty much the entirety of the time kids are working on the Desmos activity.
  11. At the end of class, if I call kids back to the main room, I remind them of anything that might be upcoming or encouraging them to see me in office hours if they were feeling lost, and then I dismiss them. I always remind them I’ll stay after if anyone needs to talk about anything, and a few times students did hang back and ask some questions to shore something up.
  12. After class ends, the first opportunity I have, I go to google classroom and think about what I saw, where students got to in the activity, and then decide what the appropriate nightly work should be. I would often have kids work up to a particular slide in the Desmos activity. I might choose a DeltaMath assignment. I might make and include a video of me working through a slide or two that kids had difficulty on the night before (if any) so they would have something to look at if they struggled.
  13. After that, when I have another small expanse of free time, I’ll look through where we are and whip up a new lesson plan for our next class based on where we got, and add it to my ongoing lesson planning document.

And the cycle starts over again the next day.

I’d use evenings and weekends to think through and create the Desmos Activities, and create videos of me working through specific slides that I thought kids would need help with. (That way after kids attempted them, I could lead them to the video and have them watch it for assistance.) (The videos were more for Algebra 2. I didn’t find I needed many of them for Advanced Precalculus since kids seemed to get the ideas fairly quickly.)

[Note: at the start of the time we had online, I would include “check in” screens to see how kids were doing/feeling. I would also reach out to individual kids if I thought something was wrong, or check in with their advisor or dean. Trying to understand and attend to the emotional well-being of my kids was really tough. But that’s a post for another time.]

 

Assessments

The assessments I gave were fairly traditional. I kept an ongoing skill list, like the one below:

Adv. Precalculus Skill List (Ongoing) – Google Docs

Then on assessment day, I would upload a test for kids to work on. I was pretty standard in terms of what the test would look like — though I was super duper extra explicit about everything in terms of how I wanted students to format their answers. (For example, I wanted the work for each skill to be written on its own page. So for a five skill assessment, they’d submit five pages.) Students were given a fair amount of time to take it on the honor system in one sitting. I didn’t have the energy to think of all the ways kids might cheat — it felt like such a low priority in terms of what I wanted to give my mental energy to. I figured it was better to just trust my kiddos, because they hadn’t given me any reason not to trust them during my time with them in-person.

Kids used the CamScanner app on their phones (they used this throughout the year to submit their nightly work, so the process was familiar to them) to submit their test on Google Classroom.

I would mark it up and give feedback using the iPad and pencil that my school got for me (bless them!), and then email it back to kids after I had marked them all up and recorded their scores.

Since we were doing Standards Based Grading, if kids didn’t show a solid understanding of the material, they had the opportunity to sign up to reassess that skill. I had a system set up that was easy to manage, but it did mean that for every test I created, I had to create two versions (one for the original go-around, one for the reassessment).

 

Feedback Loops

I was very intentional to make sure that I had a way for kids to understand what they knew and what they didn’t. Here are the ways that played out:

When given assignments on DeltaMath, if students got something wrong, they immediately know and they also are given a complete solution to the problem to learn from. The way DeltaMath is set up is that you keep working problems until you show competency — which could be doing a few problems or it could mean doing a bunch.

When given assignments on a Desmos Activity, I would go through each morning it was due and give feedback. I’ll leave no feedback on slides that kids were getting right, but on a slide where kids did a bunch of work, if they got it all right, I’d make a note of that. I’d also point out if there were mistakes. I also would have videos made (more for Algebra 2) with me working through particular key slides, so if I saw a student was struggling with something, my feedback in Desmos would include “Look at the video I created and will post on the nightly work today! I think that will help!” I would also encourage kids to meet with me in office hours to talk through things that I saw they were struggling with.

Based on looking at the whole class’s work, I would address common misconceptions or point out different interesting approaches at the start of every class.

If students messed up on a skill on an assessment and didn’t show a solid understanding, they could look at my feedback, go back to our Desmos activities, set up a time to meet with me, or talk with friends… and then ask to rework it to show a stronger level of understanding.

Close to the end of our online learning, two weeks before we ended, I asked kids explicitly about the feedback I was providing them. Here are some of their responses:

I love this structure! I love having structure in general. It’s so helpful when you go over common errors at the beginning of class, and I’m able to take notes on it. I also like the little desmos feedbacks if it was just a personal issue.

I feel as though this feedback look is extremely helpful. I particularly like the specific comments you leave on our Desmos activities – I find them super targeted and helpful. Additionally, I really like it when you share your iPad/give general feedback pertaining to the whole class in class (and sometimes start w/practice problems if you think that we need them).

It has been working really well! The comments on desmos at the beginning of class have really helped direct my questions that I ask in breakout room, and my meeting with you after school really helped me understand the material on the first test better.

I chose some of the ones that were more detailed, but almost all students said they found the feedback system helpful. It was awesome to read.

 

My Own Organization

I had everything for online learning in a single Google Drive folder that I linked to from my bookmarks bar.

13

In here, I had a folder for everything related to assessments, folders for reflection forms and feedback forms, my attendance/nightly work/check-in-with-kids spreadsheets, a google doc keeping all my individual meetings with kids and what we talked about, my ongoing lesson planning documents for both classes, and my course expectations for online learning.

I kept all attendence, nightly work, and notes on individual check-ins for students all in the same Google spreadsheet. Each got different tabs. So I would open a spreadsheet and see this at the bottom.14I didn’t want to have information spread out over a thousand documents. My goal was to be as consolidated as I possibly could be.

Five minutes before I taught each class, when Amazon’s Alexa reminded me, I opened the following windows to get prepared and ready to go:15
I’d have my google calendar up, because I often needed to refer to it to find the time the class ended. I had my ongoing lesson plan document open so I could execute the plan I came up with. I had the attendance spreadsheet ready so I could take attendance, and I had any Desmos Activities tabs open (for what kids did the previous day and/or any new activities we’d be doing).

I’ve never been a person who scheduled my life using google calendar, but during this time, I came to fully rely on it. Here’s a screenshot of what a random week looked like on my calendar:

16

 

Final Thoughts

Wow, that took longer than I anticipated to type out. I honestly figured this would just be a 30 minute blogpost where I throw up a few screenshots. Sadly I think most of this structure won’t be useable next year if we’re in hybrid mode. And I wouldn’t say it was perfect or even great. It was… fine.

The biggest thing that I felt was after a few weeks, it started to feel monotonous to me — and so I assume it was the same for kids. We’d do the same thing in most classes. I needed to find ways to break things up — different activities or ways to learn or engage with the material. But I was so fried from juggling everything and creating everything and worrying about covering key content that I didn’t have the opportunity to mix things up in the ways my kids deserved.

I should also mention that this was a lot of work that isn’t outlined here. Planning and creating the desmos activities took massive amounts of time. I had to collaborate with my teaching partner. Reach out to kids and adults when I was worried about kids. Create the skill lists and plan out the content we’d get through for the year. Write assessments and mark up assessments. Write reassessments, set them up, and mark up reassessments. Work with kids during office hours. Not to mention plan our daily advisory and attend meetings (including some of my own doing… like a book club I helped kids organize). There were many days where I’d be on my laptop every moment from 7:30am to 7 or 8pm with only a short break for lunch and dinner. Being on my laptop so long gave me headaches sometimes. Weekends were super important for me to organize myself and get as much preparation as I could for the following week. It was a lot. I found ways to make it streamlined and sustainable, but doing this work — even just “fine” work — took a lot out of me.

Distance Learning: Sorting Through It All

Technically I’m still on Spring Break, but this all ends next week, when we go back to school remotely. I’m one of the lucky few who didn’t have to get thrown into the fire immediately, so I’m using this blogpost as a way for me to sort through what I’ve done and what my take-aways are. I’ll be updating this as new things come my way, so I can keep track of everything useful in one spot.

My dear friend @rdkpickle

So my friend @rdkpickle had to start distance teaching already. She’s kinda amazing in all the ways, and so on twitter she shared out how she was doing her lessons — and noted that they’ve been going well. They are low-tech in that they use Zoom and Google Docs, and use a Google Doc as an anchor for the lesson. I love that the doc allows kids who have to miss the lesson for whatever reason (emotional/anxiety issues, having to take care of a sibling, etc.) have a way to keep up.

Before sharing it, I want to say: seeing what she did was the very first thing that I saw that made me feel like: “okay, I can do this. It’s doable.” BLESS. When talking briefly with her online, she was saying right now she couldn’t be all investigatory in the same way she was in class, almost like she was ashamed. BUT very little of we’re doing is going to be like what we do in class. The ballgame has changed (from basketball to some other sportsball!). Right now, for me, the question is can I give space and structure and community to kids where they feel they can learn a few things. And @rdkpickle’s low-tech approach allows for that!

Here’s a PDF her googledoc, which she said I could share. (And here’s the google doc.)

 

Zoom

Mike Flynn (helped by Sarah Bent) gave two wonderful webinars on distance learning that he has put online — March 11th and March 17th. (If you only have time for one, I’d watch the second one, but both are great.) They were some of the first things that made me realize distance learning was possible, by showing how to do it through his webinar. (Unlike, say, dry powerpoint lectures on teaching active learning strategies. Ahem. We’ve all been there. I just think over and over, “Physician! Heal thyself!”) My takeaways were both about distance learning and about zoom, so I’ll list them here. Fundamentally, though, the best way to learn zoom is to actually just get a few friends and all try it out together (each of y’all practicing being the leaders/hosts of the meeting).

  • If you can, start the zoom meeting 10-15 minutes early and let kids know you’ll be there. You can just have informal chats like you do before a normal class, and you can use that as an easy way to start building community.
  • You can record your sessions, but if you do that, don’t start recording during that informal chat time. (Right now, since Zoom is overloaded, it’s taking them a long time to get the recorded sessions on their website, FYI. But you can have zoom do a “local recording” on your laptop… so I was thinking if the file were small enough, I could just upload that to a google drive folder my kids could access.) Note that the chat box doesn’t show up on the recording.
  • Talk with kids explicitly about the weirdness of talking on Zoom. There are going to be awkward pauses because we can’t use facial cues and body movement to figure out if we’re going to talk or not (we’re all sort of trained to sort of check before we talk so we don’t start at the same time as someone else). So name that, and say that awkwardness is normal in zoom. You should also mention (and give) lots of wait time — just like we should be doing in our regular teaching.
  • It’s okay if you’re having kids use chat to stop every so often and take a few minutes (in silence) to read over the chat so you can respond to what you’re seeing.
  • The chat can be the “lightest lift” for interactivity, but it’s effective! One tip I got on twitter is that you can ask everyone to write a response to a question, but not press enter until you give the command. Then you’ll get a quick flood of responses that you can go through, and students can also read.
  • You can also set the zoom meeting to have the chat be private – so students are talking to you but not each other… then as you see the responses, you can say “Nice thinking, Jake!” or “If you’re thinking about a parabola instead of an exponential function, you’re going in the wrong direction!” This came directly from Michael Pershan’s experience teaching online this past week:
    ETfBLfsWkAM3His
  • If you have pre-determined questions you want to ask at a particular time during the lesson, have them written in a google doc/notepad, so when you want to ask it, you can just copy/paste them in.
  • Have everyone use their own regular names in zoom (and not emails or userhandles) to make life easier for you.
  • There is a way to include “polls” in your zoom meetings, but I couldn’t figure that feature out when trying it out!
  • You can divide your class into groups (either randomly or pre-determined) and send them to breakout rooms. You can visit any of those rooms and join in the conversations. Each breakout room is given a number when students join. You can have one person in each group (e.g. the person whose last name comes first alphabetically) to create a Google Doc in a Google Drive Folder you share with them in the chat window… And title it “Group 5, March 25, 2020.” Then all participants can write answers in their google doc and you have access to all of them in an organized way.
    • When students are first put into a breakout room, if they’re new to working with each other, start with a non-mathy but quick ice-breaker to get everyone talking (e.g. what’s your favorite pizza topping?) and build a tiiiiny bit of community before diving in.
    • SUPER COOL DISCOVERY: When I did this in Mike Flynn’s webinar, one person in my breakout room showed me a ridiculously cool feature. In any google doc, you can go to INSERT > IMAGE > CAMERA
      julie4
      And then you just take a picture of your work using the webcam, and it automatically inserts the picture in the google doc!
      julie3
      Bam!
  • Don’t go crazy with the new technology. There are so many apps and websites. Limit yourself to just a few, like two, for your own sanity and your students’ sanity. Keep it simple and easy — don’t go down the rabbit hole of looking for “the perfect way to do x, y, or z.” Be okay with the tradeoff of having “good enough.”
  • When designing online learning, start with the question “how do we want our students to learn?” Then choose your technology based on that.
  • Screensharing is awesome (so you can set up a google slideshow, and in zoom you can screenshare that slideshow to the kiddos… And you can show kids how to annotate so individuals or the whole class and write/type/draw on a screen you’re sharing (and you can save that).

 

Desmos Activity Builder 

Julie Reulbach led a webinar on using desmos for assessments, but basically she outlined all the ways we could create activity builders to actually teach content also, and bring students along with us as they navigate the pages, and we talk through what they’re doing. Her resource page is clipped below so you can see what’s there…

julie1

But importantly, her page includes links to various activity builders where you can simply copy and paste! Here’s how you copy and paste screens from better activities that your own into your own! They can even have computational layer in them!

Some key tips for creating Activity Builders (but not necessarily for assessments in particular):

  • Steal steal steal screens from other activity builder assessments if you’re doing anything fancy (e.g. self checking, anything with computation layer), because there’s no need to reinvent the wheel right now. Julie has curated a whole list of activities that she takes screens from! And desmos has curated a bunch of starter screens(e.g. “graph how you’re feeling today?”) that you can take!
  • DESMOS NOW ALLOWS FEEDBACK – so you can write a note to individual students.
    https://www.youtube.com/watch?v=P-ebHOily6k&feature=emb_logo
  • Importantly, let’s say kids are doing a desmos activity or assessment, and you want them to show their work that they’ve done on paper. All you need to do is create a screen which has a blank graph, and instruct kids to insert an image (see sidebar instructions in the image below) and they can actually INSERT a picture of their work! The workflow is a little clunky because they have to take a picture on their phones and then email/airdrop it to their laptops, and then select that file. But it took me only like 20 seconds after I had done it once.
    julie2

Some key tips for using Desmos for assessments:

  • Have kids log in with their name, but “last, first.” That way when you grade their online assessment, you can sort the responses by their username, and that will match your gradebook.
  • Have a fun introductory “hi there!” screen
  • Have students fill out an honor code/statement screen first if doing a formative assessment
  • After you finish the activity, have two screens at the end. First, a feedback screen so you can find out how they felt it went. Second, a screen asking them if they have any questions or anything they want you to know.
  • If a formal assessment, you should PAUSE the activity at the end — so kids can’t go back and change their answers or share the class code with other kids

 

Michael Pershan’s blogpost

My friend Michael Pershan has been in the thick of online teaching. He wrote a detailed blogpost about what he’s discovered thus far. I highly recommend reading it! Big takeaways:

  • His school is using Google Classroom (like ours does), so he’s using that to create a system of organization for the kids, with instructions given day-by-day (within a unit):
    He noted: “The most important thing, though, is that each learning activity becomes its own “assignment.” During week 1 I was creating large documents that students were working on over multiple days. This was good in one sense, because I had to post only one thing. But it became very difficult to monitor the progress of kids through the assignment at all. And then it became tricky to modify the plan in the middle of the week by adding on other bits of classwork.”
  • He’s using google classroom to teach kids how to upload their written work. (Note: my kids always submit PDFs of their work on google classroom, so they’re very familiar with this!)
  • To give feedback on google classroom: “Google lets you comment on the work itself via highlighting and commenting, but I’ve found it more useful to give a quick written comment that appears under the assignment itself.”

 

Twitter

Lots of great things being shared on twitter. It was so overwhelming that I stopped looking at twitter for a while, but I did save a few things:

https://twitter.com/rebeccajrucker/status/1239310493791240198

https://twitter.com/JennSWhite/status/1239963029233496066

https://twitter.com/MrCBRobinson/status/1240642486286123008

 

What Wasn’t That Useful For Me – But Here are the Nuggets I’ve Taken Away From These

What I have below doesn’t mean these aren’t good for others. It just means that for me, I like to jump in and these things didn’t quite pan out fully.

Alice Keeler had a webinar (“Oh Crap, I’m Teaching Math Online Now“) that wasn’t crazy useful for me because it was a brief overview of many things I already knew about. It was just super tech happy (look at Pear Deck! Look at Geogebra! Look at Desmos! Look at …) and didn’t give me the focus or vision I’m searching for.

Global Online Academy (GOA)’s 1 week course on Designing for Online Learning. Since this was designed to be “big picture” (so it can accommodate people from many schools and teachers of all stripes and many disciplines), I had trouble getting specifics that I wanted to latch onto. Here’s what I did get:

  • They recommended Loom for laptop screen recording, if you were going to be making videos from your laptop. It seemed pretty seamless and easy to use, based on this short video tutorial:
    https://www.youtube.com/watch?v=OvRVJ46ffoQ
  • The basics of good teaching are still important — clarity and being organized is paramount. Specifically for online learning, they highly recommend:
    • building routines early (just like with regular teaching!)
    • share the “learning goals” at the start of each lesson explicitly
    • don’t get over-excited and share too much… curate what you share and make it super easy to follow
      • using a lot of whitespace and images
      • don’t include anything that isn’t super important — focus on key ideas
      • not using too many fonts
      • everything you share with your students should be “crisp” and “clean” (not “busy”)
  • Be present for students. Create or adopt an online persona. Don’t leave them hanging, but show them continual engagement so they know you’re with them on this journey.