In Calculus, I sound like a broken record. Each time we learn something new, I say “take what you don’t know and turn it into what you do know.” I say that at least three times a week. I said it last week when doing integrals like:
We don’t know how to deal with that, but we do know how to deal with
So let’s try to turn what we don’t know how to do into something we do know how to do. For those who haven’t taken calculus for a while, the integral above is . So to do the original problem, we want to somehow get the original integral to look like
— the integral of 1 over something squared plus 1. So we rewrite the integral as
. That’s much closer to what we want to get — it looks more like something we know how to deal with. Next we use
-substitution to finish this beast off (
) to get
. Now we have something we know how to deal with, from something we didn’t.
Again today, I showed my students how to solve , and told them to solve:
. At first sight, they recoiled, but again, we used the mantra of “take what you don’t know and turn it into what you do know” to solve it. If it looks scary, fine, have a moment of panic, but then ask yourself “what does this look like” and “can I turn it into that with some simple manipulation”?
I was thinking today how this actually could be my refrain in Algebra II also. Example: I could frame quadratics in that way. Students know — or quickly learn — how to solve equations like (hopefully). But what about something like
? It’s not nearly as easy. But then we can talk about if there is a way to that what we don’t know (that equation) and turn it into something we do know how to solve (
). It’s not that I don’t do this already, but I am not always explicit about it. It is not my mantra.
But it should be. It’s how we solve math problems. We have something we don’t initially know how to do. And we have to figure out if we can simplify/rewrite/re-envision it to bring it to a place where we know how to do it.It seems stupid and simple and obvious, so much so, that I don’t say all the time. But if I started saying that as my refrain, if students really saw that math is simply this simple process, it might stop seeming like a huge bag of tricks that never fall together. They might see it as the art that it is — where there is creativity in deciding how to get from point A (hard problem) to point B (simple problem they know how to do). And all the specifics that we do in class are giving them the tools which they can use to chisel out a path from A to B. It might finally be us always trying to work out the puzzle: what does this look like that we know how to do, and can we get it to that place?
In other words, we’re now talking processes instead of methods. We’re talking problem solving instead of rote memorization. And whenever a student is stumped on a problem, you can stimulate his/her thought process by saying “we’ve always taken what we don’t know how to do and turned it into something we do know how to do… what similar things does this beast remind you of?”
So yeah, it’s not a huge revelation or anything. But I’m thinking that it might be a really amazing experiment to frame my Algebra II and Calculus classes with this mantra next year. Heck, maybe even in the next few weeks when I’m teaching exponential and logarithmic functions! I mean, yeah may look ugly. But is there a way to turn it into something we do know how to do? Namely something of the form
? Obvi.
Yes, yes, and hell yes.
I haven’t gotten it boiled down to mantra-size yet, but I do say this often to my classes. Come to think of it, I remember when I interviewed for my current position, I taught a Geometry class the Law of Sines, and I did exactly this. “You’ve only been using trig functions with right triangles. THIS is not a right triangle, so we can’t use trig on it as-is. But perhaps we could CREATE some right triangles…” I think I even may have said something like, “This is what we do in math, we take a problem we don’t know how to do, and turn it into one we DO know how to do.”
So you can imagine how this post resonated with me!
I also find myself saying similar things to my classes. I think you are dead on when you say “It’s how we solve math problems”. During a calculus review the other day, I told the students that the main difference between people who struggle with a topic and those who don’t is not usually a matter of the latter group knowing more rules or formulas or what not. Instead, it’s that the people in the second group are much more adept at recognizing how they can turn a seemingly difficult problem into one they know how to solve.
Brilliant post. You should send a condensed version into the I Want To Teach Forever blog’s “52 Teachers, 52 Lessons” contest. It is awfully inspiring.
I totally agree with you — the essence of problem solving is fully understanding the unknown in the problem and exploiting all connections to the known.
The one possible (probable?) pedagogical issue here is that students do tend to lock in on methods rather than processes at any available opportunity, and framing math problem solving as turning one thing into another will sound temptingly methodical to students. When you ask them to solve log(2x+1) + log(x-1) = 2 and say “turn it in to what you do know”, what may students will start doing is trying anything that looks like a method that could possibly bring a quick end to the problem. You know what will happen: The student will say something like:
log(2x+1) + log(x-1) = 2 -> log((2x+1) + (x-1)) = 2 [factoring out log]
-> log(3x) = 2
-> 3x = 2/log [Has the pain started yet?]
Therefore x = 2/(3 log).
Then the student will say, “Why are you throwing a fit at me? I’m doing just what you said — turning it into to something I do know!”
So I think you’re right, mathematical problem solving has to be thought of more in terms of process than method — but method is and will always be really central to understanding the process. Knowing what methods are true and which ones are false, and which ones work better in various situations, is a precondition for understanding process.
You are channeling the master! Your post reminded me so much of this passage that I had to go look it up:
I love this. If we do a good enough job of scaffolding the material students would naturally see this. Man, if it were just that easy!
Thanks y’all. It seems like my feeling about how I could frame what we do resonated with you — which means I’m probably doing something right. I’d love to take a few days this summer and see if I could map out the Alg II or Calculus curriculum based on this one premise. Can we see a direct line, for example, between solving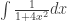 and what we did earlier in the year? I think I’d argue yes. A basic lineage would look like:
and what we did earlier in the year? I think I’d argue yes. A basic lineage would look like:
complicated integral –> simpler integral via u-substitution –> integration as antidifferentiation –> differentiation –> limits.
Do they see how much they’ve done — taking what they don’t know and turning it into something they do know? I have to highlight this more – make it more fundamental to how I frame what I’m teaching.
@RobertTalbert: point (“Knowing what methods are true and which ones are false, and which ones work better in various situations, is a precondition for understanding process”) definitely taken.