I was in the middle of writing how I made the transition from Anti-Derivatives to Integrals, and then I got swept up with school things. So a month and a half later, at the start of my summer vacay (!), I’m going to write up the very last post in this installment, where I make The Final Turn.

As a recap: I taught anti-derivatives as “the backwards question” of derivatives, but didn’t give them any meaning other than a mathematical puzzle. And my first post (Part I) starts when I tell kids we’re going to put anti-derivatives aside for a while and we’re going to think about a road trip. In this, students recognize that velocity graphs can tell you about displacement, and we are forced to contend with left handed and right handed riemann sums. The conclusion is that “the displacement of an object can be calculated by estimating the area under a velocity graph.” Importantly, for kids, this has nothing to do with anti-derivatives. In my second post (Part II), students explore this idea in more detail. They are given a bunch of step functions for velocity and they come to recognize the difference between distance and displacement, and also why we need to specify a starting position to know an ending position. They also see the need for negative signed area to represent moving backwards. Again, this has nothing to do with anti-derivatives.

This is where our story begins. The final part of this tale, where we connect signed areas (which students are comfortable with) to anti-derivatives in such a way that the connection makes sense and is actually kinda obvious. That was my goal, anyway.

At the end of the same packet students were working on in Part II, I gave the following velocity and position graphs. Like my students, you can probably see that the velocity graphs are approximations of “nice” curves (e.g. quadratic, square root, linear, sine, exponential, cubic).

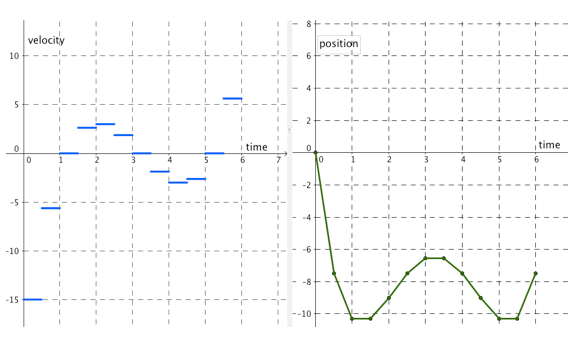
After being asked to identify some specific things on each graph (find what happens to the corresponding position piece when the velocity was greatest… find what happens to the corresponding position piece when the velocity was zero… etc.), I asked students to write six different things they noticed/observed. Kids came up with great observations! Here are some of them taken from their nightly work (note: “palley” is our class terminology for peak or valley):

• Zero on the velocity graph yields a slope of 0 on the position graph (the object didn’t move for that time period)
• Most positive value on the velocity graph yields the largest positive slope on the position graph
• Most positive value on the velocity graph corresponded with the largest change in position (from low to high) on the position graph
• The position graph usually has one more palley than the velocity graph (when we’re dealing with polynomial velocity graphs)
• When the velocity graph is negative, the position graph is decreasing
• When the velocity graph is positive, the position graph is increasing
• For the 4th graph, the velocity graph looks like a sine graph and the position graph looks like a cosine graph
• Many of the relationships between graphs look similar to derivatives we’ve been studying
• At the point of highest velocity, the position graph usually has a palley
• The area under the velocity graph gives the height of the position graph
• Velocity graph is the derivative of the position graph

Ooooh! I just found the smartboard page where we codified these in one class:

To debrief this, I had kids call out their observations and we talked about them/refined them using the graphs I was projecting at the front of the room. The most important thing I remember doing was making sure we started with “simple” observations and only then had the class move to more “complex” observations. That way kids who only noticed basic things would have a chance to voice their thinking.

From this we finally got to the conclusion we were looking for: the velocity graph is the derivative of the position graph. I had to nudge kids to make the counter-observation they get for free: the position graph is the anti-derivative of the velocity graph.

We saw all the things we had previously learned when studying the shape of a graph come out (e.g. when the derivative is negative, the original function is decreasing; when the derivative was zero, the original function often had a “palley”). Everything fit together!

We then carefully looked at small pieces of one of the graphs and discussed what we saw going from left to right, and also going from right to left.

 

This slideshow requires JavaScript.

From left to right: we saw that the signed area under the curve on the left corresponded to the change in position on the right.

From right to left: we saw that the slopiness of the curve on the right corresponded with the value/height of the function on the left.

So we codified this. But importantly, we finally got to say “antiderivative” but with meaning. HERE’S THE KEY TURN: We knew that the derivative of position was velocity. That means by definition, the anti-derivative of velocity was position (ok, technically displacement, but let’s not cut hairs right now). But we already knew from our work previously that to get from velocity to position, we had to find signed areas. THUS, accumulated signed areas and anti-derivatives were one and the same!!!

In one class, we outlined this as such.

 

This slideshow requires JavaScript.

In my other class, I had us codify things more formally.

Both ways of codifying this knowledge seemed to make sense for kids. And to be perfectly honest, the fact that antiderivatives were accumulated signed areas was always a bit magical to me. I know I truly understand something conceptually when something magical/mysterious changes and becomes a “well, duh, of course it has to be that way.” I don’t think in all my years of doing and teaching calculus that I had ever totally gotten rid of that feeling of magic/mystery until this year, when I really changed how I approached this with my kids.

Now I know you might be saying: but Sam, you never PROVED this relationship, that an antiderivative of a function and the signed area under that function were the same. Agreed. I wanted to have kids see and make sense of this relationship first. But I did do a basic proof of this (that the antiderivative of a function is the same as the accumulated signed area of that function) soon after. If you’re dying to know what came after, I had kids use a Riemann Sum applet (I slightly modified this one to not have the integral symbol on it or the “show actual area” button on it) to fill this sheet out [docx version: 2018-05-03 Riemann Sums]:

And at the very end of this packet is our “proof”:

Now this page/proof should have appeared super familiar to my kids. We actually had made this exact same argument earlier when seeing the derivative of the volume of the sphere is the surface area of the sphere, and when seeing the derivative of the area of a circle is the circumference of the circle (see post here). Although this proof did require us to work together as a class with a bit more handholding than I would have liked, it went pretty well.

And with that, I can put this three part beast to bed. If nothing else, this series just reminds me how much I really think about how students build and construct knowledge. It is something that I think about so naturally when designing new materials, but to explain all that thinking and how it unfolds in the classroom takes way more words than I anticpated! No more three part blog post series for a while!

Fin.